William Dalrymple (historian)
The Mathematics Portal
Mathematics is the study of representing and reasoning about abstract objects (such as numbers, points, spaces, sets, structures, and games). Mathematics is used throughout the world as an essential tool in many fields, including natural science, engineering, medicine, and the social sciences. Applied mathematics, the branch of mathematics concerned with application of mathematical knowledge to other fields, inspires and makes use of new mathematical discoveries and sometimes leads to the development of entirely new mathematical disciplines, such as statistics and game theory. Mathematicians also engage in pure mathematics, or mathematics for its own sake, without having any application in mind. There is no clear line separating pure and applied mathematics, and practical applications for what began as pure mathematics are often discovered. (Full article...)
Featured articles –
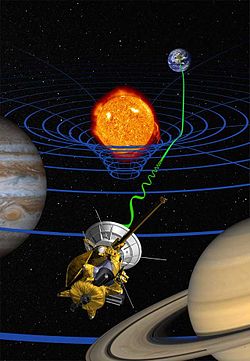
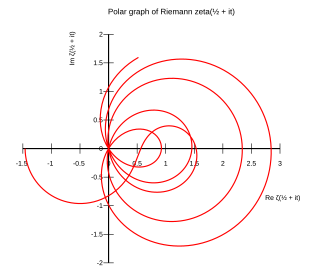
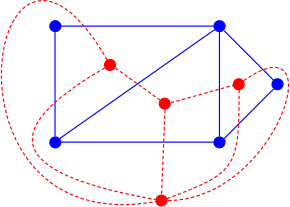
Selected image –
Good articles –
Did you know (auto-generated) –

- ... that the music of math rock band Jyocho has been alternatively described as akin to "madness" or "contemplative and melancholy"?
- ... that the British National Hospital Service Reserve trained volunteers to carry out first aid in the aftermath of a nuclear or chemical attack?
- ... that people in Madagascar perform algebra on tree seeds in order to tell the future?
- ... that in 1967 two mathematicians published PhD dissertations independently disproving the same thirteen-year-old conjecture?
- ... that the discovery of Descartes' theorem in geometry came from a too-difficult mathematics problem posed to a princess?
- ... that mathematician Daniel Larsen was the youngest contributor to the New York Times crossword puzzle?
- ... that more than 60 scientific papers authored by mathematician Paul Erdős were published posthumously?
- ... that The Math Myth advocates for American high schools to stop requiring advanced algebra?
More did you know –

- ...that a monkey hitting keys at random on a typewriter keyboard for an infinite amount of time will almost surely type the complete works of William Shakespeare?
- ... that there are 115,200 solutions to the ménage problem of permuting six female-male couples at a twelve-person table so that men and women alternate and are seated away from their partners?
- ... that mathematician Paul Erdős called the Hadwiger conjecture, a still-open generalization of the four-color problem, "one of the deepest unsolved problems in graph theory"?
- ...that the six permutations of the vector (1,2,3) form a regular hexagon in 3d space, the 24 permutations of (1,2,3,4) form a truncated octahedron in four dimensions, and both are examples of permutohedra?
- ...that Ostomachion is a mathematical treatise attributed to Archimedes on a 14-piece tiling puzzle similar to tangram?
- ...that some functions can be written as an infinite sum of trigonometric polynomials and that this sum is called the Fourier series of that function?
- ...that the identity elements for arithmetic operations make use of the only two whole numbers that are neither composites nor prime numbers, 0 and 1?
Selected article –
 |
| The German Lorenz cipher machine, used in World War II for encryption of very high-level general staff messages Image credit: Matt Crypto |
Cryptography (or cryptology) is derived from Greek κρυπτός kryptós "hidden," and the verb γράφω gráfo "write". In modern times, it has become a branch of information theory, as the mathematical study of information and especially its transmission from place to place. The noted cryptographer Ron Rivest has observed that "cryptography is about communication in the presence of adversaries." It is a central contributor to several fields: information security and related issues, particularly, authentication, and access control. One of cryptography's primary purposes is hiding the meaning of messages, not usually the existence of such messages. In modern times, cryptography also contributes to computer science. Cryptography is central to the techniques used in computer and network security for such things as access control and information confidentiality. Cryptography is also used in many applications encountered in everyday life; the security of ATM cards, computer passwords, and electronic commerce all depend on cryptography. (Full article...)
| View all selected articles |
Subcategories

Algebra | Arithmetic | Analysis | Complex analysis | Applied mathematics | Calculus | Category theory | Chaos theory | Combinatorics | Dynamical systems | Fractals | Game theory | Geometry | Algebraic geometry | Graph theory | Group theory | Linear algebra | Mathematical logic | Model theory | Multi-dimensional geometry | Number theory | Numerical analysis | Optimization | Order theory | Probability and statistics | Set theory | Statistics | Topology | Algebraic topology | Trigonometry | Linear programming
Mathematics | History of mathematics | Mathematicians | Awards | Education | Literature | Notation | Organizations | Theorems | Proofs | Unsolved problems
Topics in mathematics
| General | Foundations | Number theory | Discrete mathematics |
|---|---|---|---|
| |||
| Algebra | Analysis | Geometry and topology | Applied mathematics |
Index of mathematics articles
| ARTICLE INDEX: | |
| MATHEMATICIANS: |
Related portals
WikiProjects
![]() The Mathematics WikiProject is the center for mathematics-related editing on Wikipedia. Join the discussion on the project's talk page.
The Mathematics WikiProject is the center for mathematics-related editing on Wikipedia. Join the discussion on the project's talk page.
In other Wikimedia projects
The following Wikimedia Foundation sister projects provide more on this subject:
-
 Commons
Commons
Free media repository -
 Wikibooks
Wikibooks
Free textbooks and manuals -
 Wikidata
Wikidata
Free knowledge base -
 Wikinews
Wikinews
Free-content news -
 Wikiquote
Wikiquote
Collection of quotations -
 Wikisource
Wikisource
Free-content library -
 Wikiversity
Wikiversity
Free learning tools -
 Wiktionary
Wiktionary
Dictionary and thesaurus
More portals
- ^ Coxeter et al. (1999), p. 30–31; Wenninger (1971), p. 65.

 Read
Read
 AUTHORPÆDIA is hosted by Authorpædia Foundation, Inc. a U.S. non-profit organization.
AUTHORPÆDIA is hosted by Authorpædia Foundation, Inc. a U.S. non-profit organization.